02.03 How do I solve more complex problems mathematically?

(approximately 5 minutes exploring the Geogebra app, 10 minutes reading and a 10 minute video)

Clicking on the following picture will take you to a Geogebra site where you will be able to interact with this Rollercoaster. Click on the box with a dot and the letter A to move the points and watch the equation change.

For an introduction to why polynomials are useful, read through this Prezi presentation [website]: click on the Play button on the slide at the top right of the screen. You have already been using polynomials in the previous set of tasks. In preparation for graphing polynomials, you need to understand how to simplify them. The combination of graphing simplified polynomials is the basis for solving many complex problems, which is illustrated beautifully in this youtube video including a clip from the movie Hidden Figures, with an introduction by Professor Alan Garfinkel and an interview with the real Katherine Johnson (watch at least 4 minutes of the 9:30 minute video. Note that although this is now done by computers, someone still has to program that computer to do the math.

02.03.01 What do I already know about polynomials?

(approximately 1 minute of reading and 10 minutes of practice)

Definitions:

variable: letter that represents a number (eg. x, y, a, . . .)
coefficients: the number that multiples the variable(s) (eg. 7 in 7xy)
terms: variable(s) and coefficient separated by subtraction or addition and not within brackets
constant: a term without a variable – just a number (such as 4 or -3)
simplify: to reduce or condense a math statement to its lowest and/or shortest equivalent form
expression: a math statement of numbers and possibly variables without an equals or inequality sign, which may require simplifying
algebraic expression: any expression including variables
equation: a math statement of numbers and possibly variables with an equals or inequality sign, which may require simplifying and requires solving

Test yourself using these flashcards [website].

02.03.02 What are polynomial expressions?

(approximately 1 minute of reading, 2 10 minute investigations, and 10 minutes of definition practice)
What is the definition of polynomial expressions?
Compare the following list of polynomial expressions and non-polynomial expressions and determine a definition for polynomial expressions. Check your definition against the provided definition under the “i”. (Note: the following interactive may take extra time to load.)

What is the degree of a polynomial expression?
Compare the following chart of polynomial expressions determine a definition for a polynomial expression degree. Check your definition against the provided definition under the “i”. (Note: the following interactive may take extra time to load.)

Why is knowing the degree important?
The degree determines the most number of solutions for that function which informs you how many times it will cross the x-axis when graphed.
Knowing the following definitions can also be helpful.

leading term: the term containing the highest power of the variable or the term with the highest degree
leading coefficient: the coefficient of the leading term.
degree 0: a nonzero constant
degree 1: a linear function
degree 2: a quadratic function
degree 3: a cubic function
degree 4: quartic or biquadratic

Practice finding the degree of an expression on this website (8 questions).

If you are still unclear and want further explanation, read this website which explains how the graphs look.

02.03.03 How do I multiply polynomials like in the Geogebra introductory activity?

(approximately 3 hours of reading and practicing in total)(Video shows how to simplify polynomials using Algebra Tiles)

In order to get to the point where you can graph a polynomial, you must be able to multiply its components and divide it into its components. There are a few different methods for multiplying polynomials.

One idea you need to remember is that manipulating terms is no different than manipulating numbers.

(2)(3)(4)=(6)(4) or (2)(12)

but not (2*3)(2*4)=(6)(8)

2a*3b=6ab

but not 6a*6b=36ab

AND

(2)(3+4)=(2)(7)=14 or (2*3)+(2*4)=14

but not (2*3)(2*4)=(6)(8)=48

(2)(a+b)=2a+2b

but not (2a)(2b)=4ab

Listen to the online videos and do the exercises in this Khan Academy unit. (approximately 40 minutes total)
Read through this OwlCation [web page] article and do the exercises which includes the FOIL method and the grid method. (approximately 40 minutes total)
Use this NCTM (National Council of Teachers of Mathematics) online interactive to multiply using Algebra Tiles. Click on the ‘Expand’ tab to practice multiplying polynomials. Click on the paper icon to change to a suitable question for your level of understanding. (approximately 40 minutes total – try to do 20 minutes at 2 different times)
There are special products which can can be done traditionally, but can have a faster solution time if you understand them. Listen to the online videos and do the exercises in this Khan Academy unit. (approximately 20 minutes total)
A good test of your understanding through 10 questions can be found at this MathBitsNotebook website. (approximately 20 minutes total)
Further practice – create some examples for yourself and use this online polynomials calculator to check your work. (approximately 40 minutes total)

02.03.04 How do you calculate gardening needs?

(approximately 2 minutes reading and 20 minute activity)

“Mom’s Vegetable Garden” by Chiot’s Run is licensed under CC BY-NC 2.0

Your parents want to refresh all the garden beds. They want to add 8 cm of mulch to all the flower beds and 5 cm to the garden beds. You decide it would be a lovely combined Mother’s and Father’s Day gift if it isn’t too expensive. You know they plan to get Magic Mulch from Victoria Landscape [website] because the garden beds just need a slow release fertilizer to mix in. Based on information from a number of articles, including this one on lasagna gardening [website], they are going to cover the soil of the flower beds with one layer of cardboard and then put down the mulch to decrease the weeds. How much mulch would you need (it is sold in cubic yards which is 3 feet by 3 feet, and each foot has 12 inches and each inch is 2.54 cm)? The garden isn’t a plain rectangle so you can’t use the calculator on the Victoria Landscape website. The three garden beds are the same size. To top it off, you have measured based on two toys in the backyard (a bat and an airplane) since you can’t find a measuring tape and don’t want to give away your surprise by asking for one. Can you create your final equation? Then you could estimate the values for ‘a’ (airplane) and ‘b’ (bat) to see if it isn’t too expensive a gift, but still have the correct statements to calculate the actual needed amounts.Use the Hint and Checkpoints to check you are on the correct path as you go. 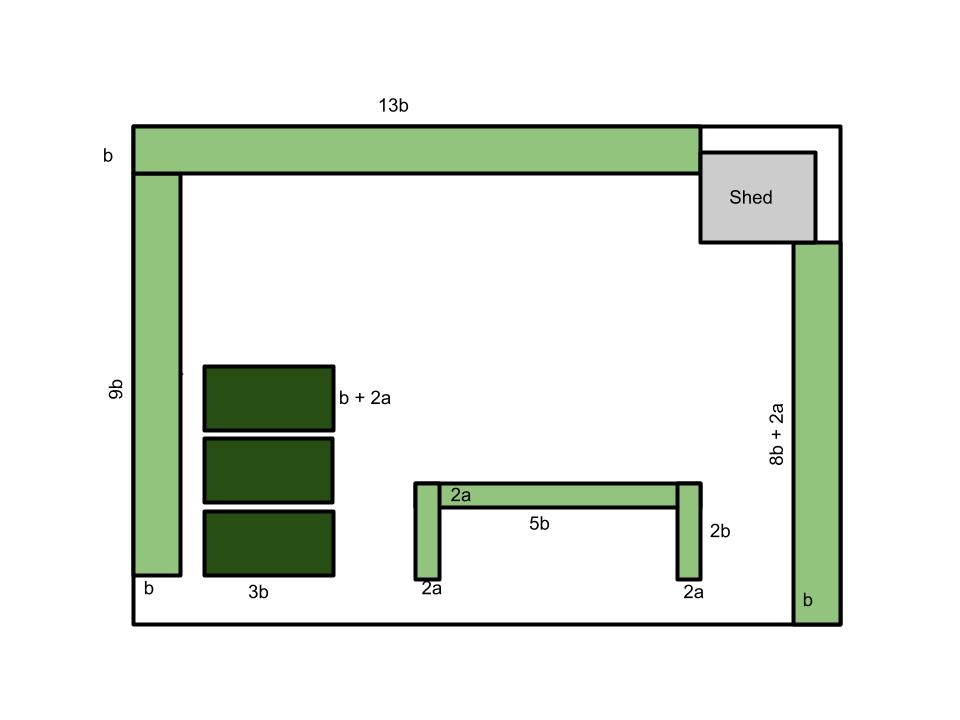Drawing of Backyard

Hint 01 Initial Step

calculate the surface area of flower beds and garden beds separately

Checkpoint 01: surface area calculations

When calculating your mulch needs, convert your depth of mulch from cm to inches to start.

Checkpoint 02: volume calculations

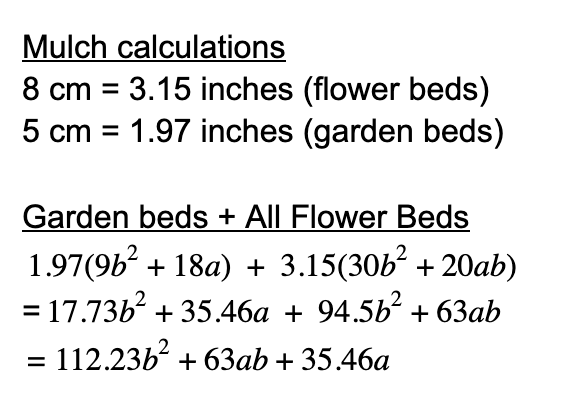This is in cubic inches.You estimate the bat to be 30 inches long and the airplane to be 10 inches long. How many cubic yards of mulch do you need? Is that too much to pay for a Mother’s and Father’s Day combined gift for your parents?

Checkpoint 03: estimated value

02.03.05 How would I do on a polynomial test?

(approximately 30 minutes)

This quiz has 4 levels of questions: simple, more complex, challenging and challenging in an unfamiliar situation.

Access the Polynomial Quiz Google document or download the Polynomial Quiz pdf.

Download the Polynomial Quiz Key or access the Polynomial Quiz Key Google document.

For Teachers: